In attempting to arrive at a fair critique of Alexander de Seversky's "Combat Plane" concept, I discovered something unexpected about the "Combat Box" formation developed in late 1942 by then-Colonel Curtis LeMay.
In particular, the numerical and spacial organization of the Combat Box involves the repetition of a single fundamental structure in three-dimensional space to build larger and more complex structures which, in turn, recapitulate the spacial form of the fundamental structure. In other words, Col. LeMay's geometric concept is fractal.
Obviously, dire operational necessity in a lethal environment dictates that perfection in fractal geometrical form not be a priority, but just look at how close this formation comes to such perfection:
Standard Group Combat Box Formation of 20 Aircraft - August 1943
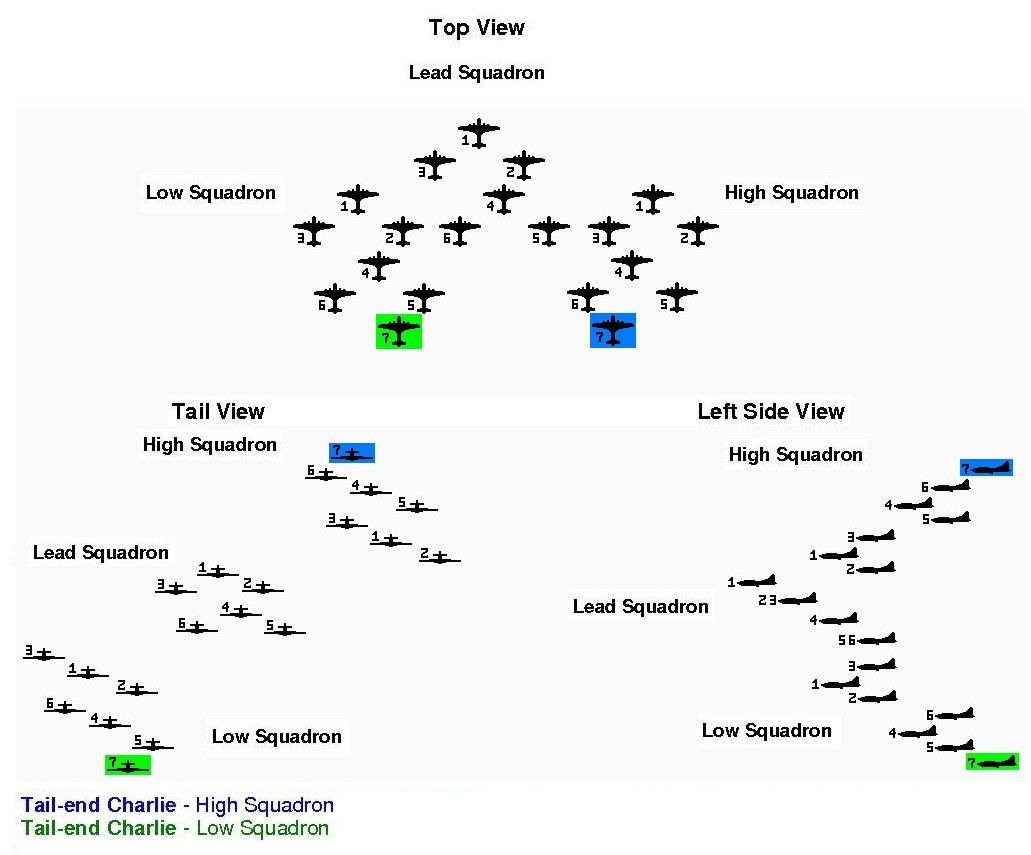
from:
http://www.303rdbg.com/formation.htmlObviously, a more perfect fractal form would require a nine-plane squadron of three flights of three aircraft each, as part of a three-squadron group of 27 aircraft, with no Tail-End Charlies to protect the swallow-tail-shaped opening at the rear of the box from enemy fighters attempting to break up the formation from six o'clock level, but, once again, wartime necessity obviously militates against such theoretical perfection in favor of operational practicality. But the basics of fractal geometry are clearly present in Lemay's ideas, much as Pythagoras is wrongly credited with having discovered his eponymous theorem when he merely rediscovered what a group of tax-cheating Greek farmers had clearly figured out earlier.

